The Geometrical Concept of the Derivative

The Geometrical Concept of the Derivative

If you have ever found the slope of a line on a graph, that is the derivative. When we are looking at curves instead of linear graphs, it gets difficult to find the slope at every point, because the slope is constantly changing. A way to find the slope is to zoom in on the graph at a point and find the slope at that point.

A way to find the slope is using the rise over run method, or the formula for slope:

The way to get a better approximated slope, or derivative, is to make the two x values as close as possible. This is a tedious process when you want to find the slope for many points on the graph. This is where differentiation comes in. The definition of a derivative comes from taking the limit of the slope formula as the two points on a function get closer and closer together.
For instance, say we have a point P(x, f(x)) on a curve and we want to find the slope (or derivative) at that point. We can take a point somewhere near to P on the curve, say Q(x+h, f(x+h)), where h is a small value. Now we can plug these values into the slope formula:

Solving for this will get us an approximation of the slope, but it still will not get us an exact value. We want h to be as small as possible so we can get the slope at P, so we let h approach 0.

Limit Definition for the Derivative

This is the slope of the tangent line, or derivative at point P. This gives us the instantaneous rate of change of y with respect to x.
Let's do an example. Consider the function:

Then we substitute x+h in for x

Taking the limit, we would get

Now we simplify

Factor out an h

We can see as h goes to 0, we are left with 6x+2.

This linear expression 6x+2 is the derivative for the function, and we can find the slope of the tangent at any point on the curve by plugging in the x value of the coordinate.
In the graph below, the original function is red and the derivative is green.

Notice that when the slope of the parabola is negative, the function of the derivative is below zero, and when the slope of the parabola is positive, so is the function of the derivative. When the parabola dips and the slope changes from negative to positive, the function of the derivative goes from negative to positive. We can see that at f(-1), f'(-1) = -4, so the slope at -1 is -4. Similarly, at f(0), f'(0) = 2, so the slope at 0 is 2.
Though we have seen the form of the derivative using the limit, it can also be notated as dy/dx, f'(x), or y'

Different notations for the derivative

d/dx means that we are taking the derivative with respect to x.
f'(x) denotes the derivative of f(x), and y' denotes the derivative of y.

Taking the Derivative of Polynomials

Finding the derivative for some functions is harder than others, and can be a tedious process when using the slope formula. Luckily, there is an easier way of obtaining the derivative of polynomials without using limits. Newton and Leibniz discovered an easy way to find the derivative of harder functions that only takes a few steps. Let's look at an example:

The first step to finding the derivative is to take any exponent in the function and bring it down, multiplying it times the coefficient.

We bring the 2 down from the top and multiply it by the 2 in front of the x. Then, we reduce the exponent by 1. The final derivative of that term is 2*(2)x¹, or 4x.
For the second term, the exponent is assumed to be 1, so we bring it down and multiply it by the coefficient in front of the x. Then, we reduce the exponent by 1, making it 0. The final derivative of this term is 1*(-5)x⁰. Note that any number raised to the 0th power is 1, so our simplified answer is 1*(-5)*1, or -5.
The third term is eliminated because it does not have an x, which means it is a constant. The reason for this is because the number 3 can be written as 3x⁰, and when the 0 comes down the whole term becomes 0. Now we are left with our simplified derivative:

Notice that the derivative is linear and the original function is quadratic. The derivative will always be one degree less than the original function. Here is a general rule for taking the derivative of all terms of a polynomial where c is a constant:

Let's do another graphical example

Differentiable and Non Differentiable

Now, you must be careful when finding the derivative, because not every function has one. Most functions are differentiable, which means that a derivative exists at every point on the function. Some functions, however, are not completely differentiable.
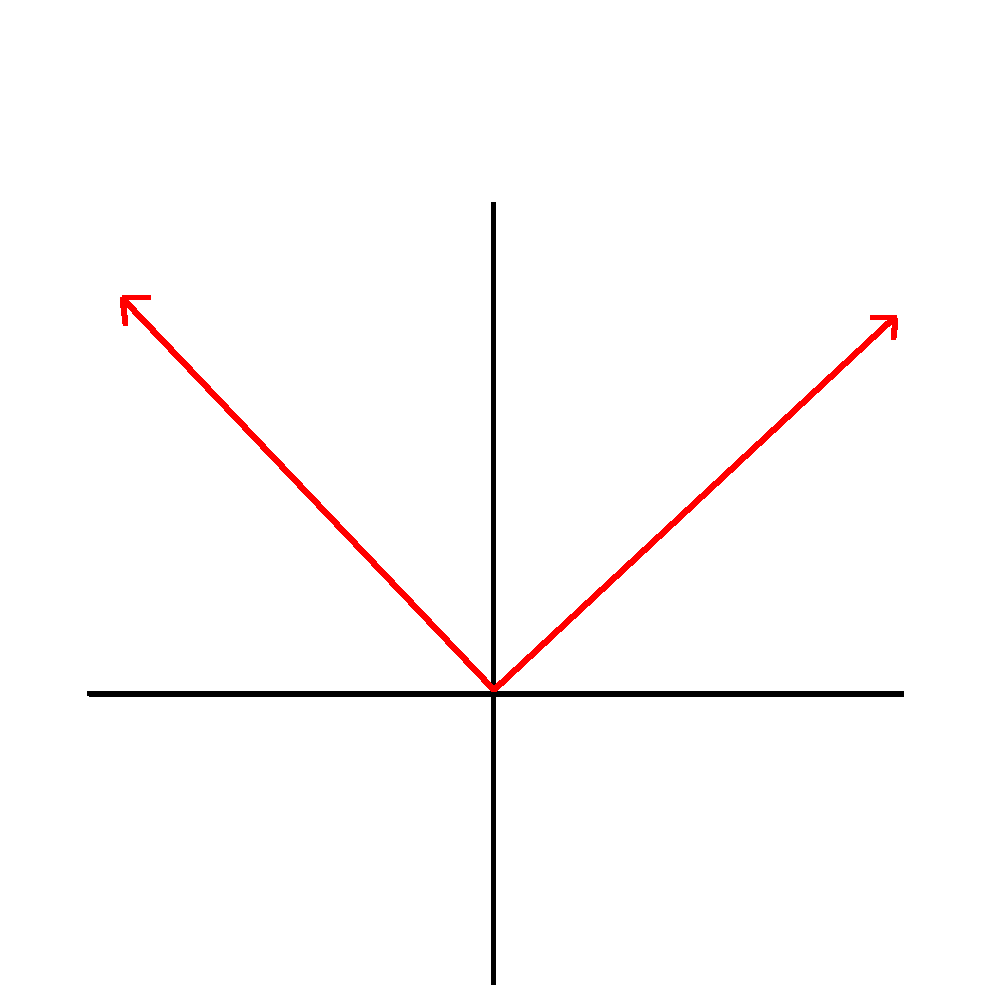
Let's find the derivative of the following function at x = 0.

The limit as h approaches 0 from the left is different than when h approaches 0 from the right. This is equivalent to saying the derivative (or slope) on the left is -1, whereas the derivative of the right side is 1. What is the slope where they meet at the origin?

Looking at the graph, we can see that at the origin there is not a definite slope because there are multiple tangents, so there is not a derivative at that point. Therefore, the function does not have a derivative at x=0, so it is differentiable everywhere except for x = 0.
We must note that in order for a function to be differentiable, it must be continuous.

Finding the Tangent Line

Earlier, we found the slope of the tangent line at a point using the limit definition of a derivative. Let's do an example finding the tangent line at a given point using the power rule for polynomials.
Find the equation to the tangent line to the graph of f(x) = x² + 3x at (1,4).

We find the derivative using the power rule for differentiation

Plug in our x coordinate into the derivative to get our slope

Now we can use point slope form to find the equation of the tangent line. (1,4) is our point and 5 is our slope

The Physical Concept of the Derivative

Isaac Newton focused on the physical concept of differentiation as it applied to mechanics and instantaneous rate of change. As it relates to mechanics, the rate of change is defined as velocity, or speed, when we are talking about distance over a period of time. Just like the geometrical approach, visualize that you are traveling from point A to point B. We use the formula for the slope to find the average velocity:

Now, if we want to find the instantaneous velocity, we want the change in time to get smaller and smaller. We introduce the concept of a limit as the change in time approaches 0. We end up with

Notice that this is the exact same as the geometric definition of the derivative, but with different variables. The physical definition is based off of the geometric definition, and all of the rules of derivatives apply to both. While you can find velocity by taking the derivative, you can also find the acceleration by taking the second derivative, i.e. taking the derivative of the derivative.
Let's do an example.
Find the velocity and acceleration of a particle with the given position of s(t) = t³ - 2t² - 4t + 5 at t = 2 where t is measured in seconds and s is measured in feet.

Velocity is found by taking the derivative of the position.

At 2 seconds, the velocity is 0 feet per second.
The acceleration is found by taking the derivative of the velocity function, or the second derivative of the position.

At 2 seconds, the acceleration is 8 feet per second squared.

Let's analyze the graph from a physical perspective. The black curve is the object's position. Notice that when the curve has a hump, the velocity function hits 0. Picture an object going a certain distance in a straight line and then coming back -- the object cannot turn around without the velocity resting at 0. This is the same for the acceleration as it relates to the velocity function. Also, when the acceleration is 0, the graph of the position function looks like a straight line around that point. This is because when the acceleration is 0, the velocity of the object is staying the same, therefore the slope will be constant.